tepary

420-69-6669

Queipo de Llano

oops! all branches!

Why can’t I hold all these continuua?!

It’s okay. The Real numbers are dense (within the real numbers), so no matter how many branches we make, we’ll always have just as many real numbers jammed arbitrarily close together on all branches!

I buy them in bulk from home despot in amerikkka

counterpoint

DAH DAH DAH

I’m the son of fabaceae

The beanis of suburbia, the legume of none of the above on a steady diet of:

DAH DAH DAH

Nitrates and urea and

compost and manure and all the nitrogen and the co2 that is in the atmospheeere

and there’s nothing wrong with me

this is how I’m supposed to beeeeaaaaaaaaaan

in a land of cereal grains that don’t believe in beanis

Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean

Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean Bean

nobody wants to work anymore

If I used my immunity-from-contracting-and-transmitting diseases wish on myself and my partner, I would mask anyways because explaining that I don’t need to wear a mask because a supernatural force granted me 3 wishes is harder than just showing people that hot gamers should mask too

^_^ thank you!

I like how compact this one is ;)

Not quite. The wording “equivalence classes of … with respect to the relation R: aRb <==> lim( a_n - b_n) as n->inf” is key.

https://en.wikipedia.org/wiki/Equivalence_class

loosely, an equivalence relation is a relation between things in a set that behaves the way we want an equal sign to

For an element in a set, a, the equivalence class of a is the set of all things in the larger set that are equivalent to a.

No. 0.99 is 0.9+0.09. The proof I gave shows that 0.99999999999999999999999999999999999(…) is equal to 1.

and wants to know why 0.999… = 1

\begin{align} 0.999… &= 9\cdot(0.1+0.01+0.001+… ) \ &= 9\cdot( 0.1^1 + 0.1^2 + 0.1^3 + … ) \ &= 9\cdot(\sum\limits_{k=1}^\infty ( \frac{1}{10^k} ) ) \ &= 9\cdot(\sum\limits_{k=0}^\infty ( \frac{1}{10^{(k+1)}}))\ &= 9\cdot(\sum\limits_{k=0}^\infty \frac{1}{10}*(\frac{1}{10^k})) \ &= \frac{9}{10}\cdot (\sum\limits_{k=0}^\infty (\frac{1}{10^k})) \ &= \frac{9}{10}\cdot \frac{1}{(1-(\frac{1}{10}))}\ &= \frac{9}{10}\cdot \frac{10}{9} = 1 \end{align}

The crux rests on a handy result on from calculus: the sum of an infinite geometric series looks likes s = 1/(1-r), when s = \sum\limits_k=0^inf r^k, and |r| < 1.

Sorry for the latex. When will hexbear render latex? This is a bit more readable:

(aesthetic edit for our big beautiful complex analysts)

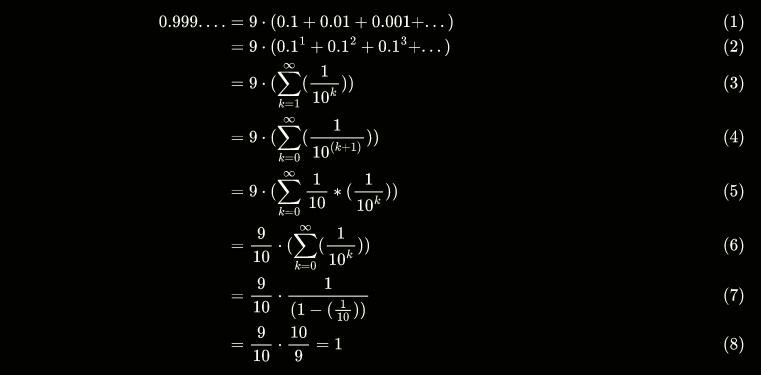
What are your top 2?

Idk. I make a different bean chili in the pressure cooker every week with whatever veggies I can get and whatever variety of beans we have on hand (which is usually a lot because I’m fortunate enough to live in a place with an absurd variety of plant agriculture). We vary the profile of the chili between a few indian-ish seasoning mixtures, this tasty african mixture with a ton of cumin and ras-al hanout, mexican-ish spices, and a few other blends. I use this to make a lot of burritos and tacos. Occasionally we’ll mix them into patties but that’s a huge chore. When my partner or I feel bored with these, we pick a place on a map and try to learn a recipe or two from there and then mix a chili with that dish’s flavors.

Aside from the chili, we’ve been making a lot of indian recipes:

this shit is amazing, but it’s a rare treat for me:

Insects also have brains. Some arachnids have the capacity for fairly complex cognition (e.g., the portia spider’s hunting behaviors, jumping spiders communicate with eachother and in my opinion, engage in playful behavior).

The issue isn’t with your terminology, but rather with what it reveals about the imprecision/inconsistency of your reasoning on these matters.

If you’re going to draw a line at eating beings that can feel the harm done to them by eating, it might serve you to explore that boundary more thoroughly.

yeah the common granulated sugar at the grocery store is usually bleached and mixed with bone char

the most fucked up thing about this in the USA is that they’re allowed to call the ingredient “natural carbon” when it’s used in a situation where it’s required to be listed

So I see no substantial moral difference between eating plants or invertebrates, for example - neither can feel harm.

octopodes have capacity to feel pain and likely experience emotion

https://www.youtube.com/watch?v=V6K1kVUct24

it does get boring pretty quick when it’s just chickpeas and beans as the base of everything.

skill issue

There are so many fabaceae that can be prepared in such a variety of ways that this should appear constructively absurd.

Another way to see it: “it does get boring pretty quick when it’s just chicken and beef as the base of everything”

bees can consent

elaborate